Steffen's Flexible Polyhedron

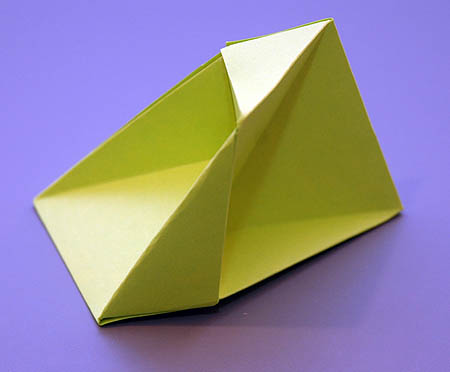
Most polyhedra are rigid. All convex polyhedra are. However, it turns out that there are truly flexible polyhedra. Early attempts at creating one resulted in polyhedra that couldn't be realized because some faces intersected each other. Robert Connelly discovered the first flexible polyhedron that doesn't have self-intersections. Klaus Steffen created this example of a flexible polyhedron with the fewest vertices possible.

Steps

1. Cut out the pattern piece.
   
2. Score the folds. The dashed lines are valley folds and the dot-dashed lines are mountain folds.
   
3. Glue the tabs as marked.
   

Notes

• A flexible polyhedron has the same volume regardless of its state of flex. Robert Connelly proved that this is true of all flexible polyhedra 1997.
  

Links

• There is an animation of Steffen's flexible polyhedron on the Wolfram Demonstrations Project website. (But making a model is better.)

References

• (Cromwell 1997)