Interesting things to make out of paper—from mathematical models to toys.
Curved Folding
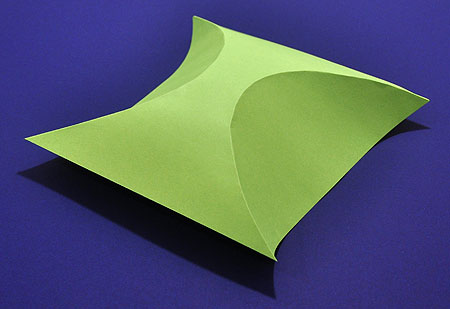
This elegant shape was formed by making two parabolic creases. That's it! The curved folds coax the piece of paper into a 3D shape.
Think about the 3D surfaces that you can make out of paper without folding. You can roll a sheet of paper into a cylinder and a cone, but try as you might, you cannot form a sphere without introducing all sorts of kinks, wrinkles, and puckers. The mathematical name for the types of shapes that you can make from paper are developable surfaces. A plane, cylinder, cone (both elliptical and circular) are examples of developable surfaces. The final developable surface is a tangent developable. To picture this type of surface think of a strip of paper that you put waves or twists into. Regular paper folding and curved folding create shapes built from developable surfaces.
Steps
- Score the crease line that is marked on the pattern and cut out the square.
- Pinch along the crease line.
Links
The project above is only a hint at what is possible with curved folding. Curvedfolding.com has photos and crease patterns for many wonderful shapes including the one shown here. A pattern for it can be found on Philip Chapman-Bell's Flickr page.
I tried to recreate this shape that Dr. David Huffman developed based on a brief write-up on his work that includes a photo. My pattern for this design is here: 8½" × 11" Pattern and A4 Pattern.- Erik Demaine has a history of curved folding on his website.
