Spherical Model - Cube

spherical model of planes of symmetry in a cube

This beautiful model is made up of 48 spherical triangles that are glued together. 48 sounds like a lot of triangles to glue, but if you follow the steps below, you can finish this project in about an hour and a half. There is no arguing that the result is a gorgeous model that helps you visualize the planes of symmetry in a cube (and in an octahedron).


Materials

  • The pattern
  • Four sheets of card stock (heavy paper), two pages each of two colors
  • Glue and a brush to apply it
  • Scissors
  • Stapler
  • Pushpin
  • Paperclips

Steps

  1. Print out one copy of the pattern.
  2. Staple each page of the pattern to two sheets of colored card stock (heavy paper) in the blank spaces between the pieces. Use a different color of card stock for each page of the pattern. Staple in many places including between pieces. This will hold the pattern and the card stock together so they don't move when you cut them. (You could simply print the pattern onto colored paper, but then the outlines will show, ruining the effect.)
  3. Use a pushpin to poke holes through the card stock where the black dots are on the pattern. This will mark your fold lines and make it easy to fold there.
  4. Cut out the patterns. You will end up with 24 pieces of each color.
  5. Fold the pieces up into triangles. Notice that the different colors will fold up to mirror images of each other.
  6. Glue the tab on the outside of each triangle. Let dry. You will now have 48 triangles.
  7. 0905-s07Glue the longest sides of one color triangle to the longest side of another. Continue to pair up triangles like this until you have 24 pairs of triangles glued together. Let dry.
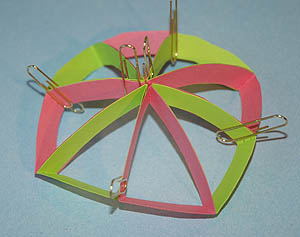  8. 0905-s08Glue four pairs of triangles together along the longer side. The colors will alternate. Repeat for the rest of the triangles. You will now have six groups of eight triangles. Use paper clips to hold the triangles together while they dry. Slide one paper clip into each corner to keep them together until they are dry.
  9. Glue the six groups together.

Notes

  • This model is based on platonic solids-in this case a cube or an octahedron-nestled inside a sphere. Each group of eight triangles that you assembled in step 8 forms a "hat" that sits on the face of an imaginary cube inside the sphere. If we assembled the triangles in a different order, we could have made "hats" for 8 faces of an octahedron. However, you might be able to imagine the octahedron inside of your completed model if you think that each of its vertices would touch the sphere wherever eight triangles meet.

References

Comments